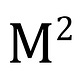Consider this comparison between a slow and a fast train. The slower train is the current train while the faster train is being proposed to replace it.
Setting aside the explicit cost of making the improvement, suppose a new train (high speed) will shorten a 5-hour trip to be just 3.5 hours. That is a significant savings in and of itself.
This isn’t just a hypothetical. It is the actual experience for the Acela Line between Boston and NYC when Amtrak started using the Acela Express in the early 2000s.1 This is the time savings achieved through the tilting advancement for this actual line.
This savings of 1.5 hours is a meaningful amount of time in both an absolute sense and a relative sense (a 30% reduction). But is it the 1.5 hours or the 30% that makes this matter? Which magnitude is meaningful?
Saving 1.5 hours off of a transatlantic cruise, which typically takes about 12 days, is not meaningful. But neither is shaving 18 seconds off of a 1-minute walk (30%) to the coffee machine at work.
Putting the savings in money terms doesn’t necessarily help at all, which shouldn’t surprise us since time cost is generally more meaningful than using a nominal or real dollar measurement. But even that can be incomplete.
I remember years ago listening to Dan Ariely on NPR (I think it was on “Marketplace”) in his somewhat regular behavioral economics segment. He was discussing his example of buying a pen versus buying a suit. The first question in his lesson was would you leave the store you are about to make the purchase of a pen for $22 if you learned that a store 15 minutes away was selling the same exact pen for $15 (a $7 savings). The second question was replace the pen with a $400 suit that you learn is being sold for $393 15 minutes away. Same $7 difference. Same distance away.
His lesson is while most people say yes to pursing the cheaper pen, they say no to leaving to buy the cheaper suit. He claims this is irrational as we should look at it as the same $7 savings.
There are lots of reasons Ariely’s thinking is incomplete or fallacious. Here are two things he neglects to consider:
Signaling—both the reputational status for the buyer as well as a proxy for trustworthiness of the seller are at play. The relatively small percentage difference in the price of the suit doesn’t give me any concern that I may not know what I’m getting. However, the large percentage difference for the pen does.
Rules of thumb that help automate decision making—we cannot spend our lives chasing every penny, dime, or dollar.
All of it circles back to if the magnitude matters, which is often times not straightforward or unidimensional. Thinking about things “on the other hand” is fruitful and legitimate. Understanding that different people in different circumstances will weigh factors differently is an essential part of good economics. It is why economics is not an exact science—if it is a “science” at all.2
Cutting in half the risk of an extremely rare cancer is not meaningful. Reducing the death rate of lung cancer by 5% would be. Again, we would need to understand the full (opportunity) cost involved in achieving the lung cancer reduction to fully appreciate the scale (magnitude) of the change, but no such calculation is probably needed in the case of a 50% decline in the risk of a six-sigma cancer—any cost including the cost of the analysis alone is probably too much.
To understand something is to understand the magnitude actually at play. Statistics may seem meaningful at first glance only to melt into obscurity when properly framed. Likewise a seemingly trivial number can take on momentous importance in the right light.
Thanks to Brian Potter’s Construction Physics for this information.
I’m a bit agnostic on this existential question.